Sound absorbing porous materials by using higher modes in acoustic metamaterials: theory and design
team: Camille Perrot (MSME), Denis Duhamel (NAVIER)
Functional materials are of major interest in creating and providing sustainable habitats. The aim of this project is to improve the material functionality for sound proofing. We plan to implement an entirely new method to enhance the efficiency of material sound absorbing property in a broad-band fashion including low frequency regime. The new method is based on employing higher acoustic cavity modes propagating in metamaterials within the linear approximation– which is fundamentally different from point-mass inclusions exhibiting non-linear geometric effects. The generalized nonlocal theory of sound propagation in porous media, which has been recently proposed and validated, provides us new physics with a deep understanding of acoustic metamaterial behavior and effective properties. This theoretical framework takes fully into account the effects due to temporal dispersion as well as spatial dispersion.
The nonlocal effects due to spatial dispersion result in accessing the macroscopic higher modes propagating and attenuating in the material. In fact, in a dissipative material, such as a rigid porous material saturated with a viscothermal fluid, sound can propagate with several complex wavenumbers. The fundamental acoustic mode is considered to be the least attenuated mode with the smallest absolute value of the imaginary part of the wavenumber. The higher modes are, therefore, those with larger imaginary part of the wave number and consequently attenuate more in the material. In addition, we precisely describe the viscous and thermal losses from the basic equations of the departure. In particular, the nonlocal theory provides us with a method to obtain the effective parameters, i.e. frequency-dependent effective density and effective compressibility, associated with the higher modes. This is an important step towards designing a new class of metamaterials based on their homogenized effective properties associated with the higher modes which has not been studied yet, and presents a very strong potential to extend the limits of sound absorbing metamaterials.
The objective of this postdoctoral research project is to find topologies enabling us to excite the second mode, in a subwavelength acoustic metamaterial, which is much more attenuated compared with the fundamental mode. The work will be divided into three parts. First, the theoretical aspect will be clarified and adapted to the specific problem considered here. Then, numerical computations will be developed to evaluate a limited number of potentially interesting cases. Finally, a sample will be built and tested to check the efficiency of this new material.
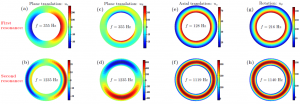
Figure 1: Displacement field distributions inside the coating layer close to the first and second resonance frequencies for the modes related to plane translation, axial translation, and rotation. The displacements are normalized such that they have fixed unit value on the outer boundary of the coating.
Publications:
[1] Favier, E., Nemati, N., Perrot, C. He, Q.-C. (2018), Generalized analytic model for rotational and anisotropic metasolids, Journal of Physics Communications 2, 035035-16 (DOI : 10.1088/2399-6528/aab5a5).
[2] Favier, E., Nemati, N., Perrot, C. (2020) Two-component versus three-component metasolids. Journal of the Acoustical Society of America, Acoustical Society of America, 148 (5), pp. 3065 – 3074. ⟨10.1121/10.0002424⟩. hal-03059349
